PROG0237 - Miscalculations of da Vinci
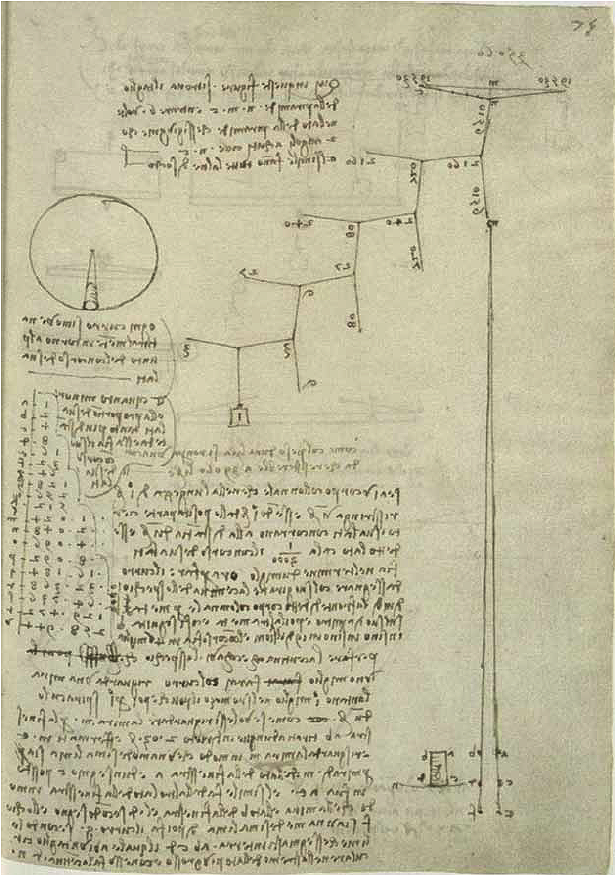
Leonardo da Vinci — the genius of the Renaissance — made some miscalculations. Not in complex formulas, but in simple sums. Below you see for example page 75R of Codex Madrid I. This collection of manuscripts dates back to the period around 1490, but it was only discovered in 1966 in the Bibliotheca National de España in Madrid together with the so-called Codex Madrid II. The two volumes, containing 197 pages, are bound in red leather. Topics discussed include mechanics, statics, geometry and construction of fortifications. There is a list of 116 books Leonardo was using at the time, including some basic Latin grammar books. The text is written in Italian dialect with some errors. The manuscripts are of great importance as they contain about 15% of Leonardo's notes referenced today, but are also important for the quality and relevance of the works they contain, which are among the major engineering treatises of their time. Nonetheless, it is less known than for example the Codex Leicester that was sold to Bill Gates at Christie's auction house on 11 November 1994 in New York for $US30,802,500.
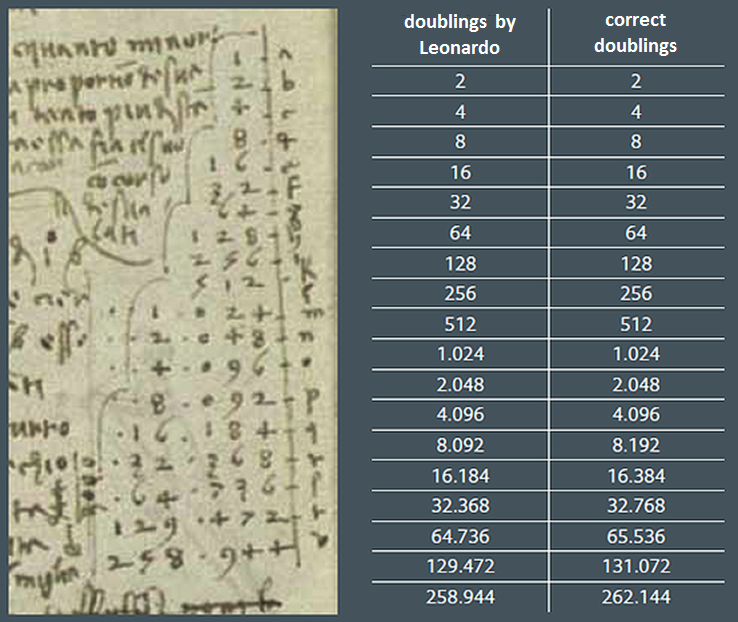
On page 75R of Codex Madrid I we take a closer look at the table on the left side of the page, somewhere in the middle below the circle or sphere. In mirrored handwriting you see a series of numbers representing successive doublings of 2. But Leonardo makes a mistake at number 4096, which he doubles to 8092 (instead of 8192). After this, the duplications are again correct, taking into account the error made earlier.
Unmistakable this is a miscalculation of Leonardo and not of some sloppy copyists, as it was found in the original (mirrored) manuscript of da Vinci himself. That it was only discovered right now, five hundred years after da Vinci's death, is probably due to the late discovery of the manuscript, barely fifty years ago.
Input
An integer $n \in \mathbb{N}$.
Output
The first line contains the letter a, followed by a space and the integer $n$. The next 25 lines each contain the next letter of the alphabet, followed by a space and the doubling of the integer on the previous line. Following Leonardo da Vinci, integers are always written in mirror writing, meaning that the digits of an integer are in reverse order.
Example
Input:
2
Output:
a 2 b 4 c 8 d 61 e 23 f 46 g 821 h 652 i 215 j 4201 k 8402 l 6904 m 2918 n 48361 o 86723 p 63556 q 270131 r 441262 s 882425 t 6758401 u 2517902 v 4034914 w 8068838 x 61277761 y 23445533 z 46880176
References
Leonardo da Vinci — het genie uit de renaissance — maakte rekenfoutjes. Niet in moeilijke formules, maar in eenvoudige sommetjes. Hieronder zie je bijvoorbeeld bladzijde 75R uit de Codex Madrid I. Deze verzameling vellen dateert uit de jaren 1490, maar werd pas ontdekt in 1966, samen trouwens met de zogenoemde Codex Madrid II. Er staan voornamelijk uitgewerkte tekeningen in van allerlei mechanismen, met een korte bespreking. Sommigen denken dat het Leonardo's bedoeling was deze bladzijden te verwerken in een afzonderlijk boek over het ontwerpen van machines. Anderen menen dat de codex paste in Leonardo's plan om een grootse encyclopedie te maken op basis van tekeningen. Hij koesterde dit idee zijn hele leven, en misschien passen ook andere geschriften van hem hierin: er zijn ongeveer 7.000 bladzijden van zijn hand bekend. Een beroemdere codex is die van Leicester, waarvoor Bill Gates in 1994 30,8 miljoen dollar neertelde.
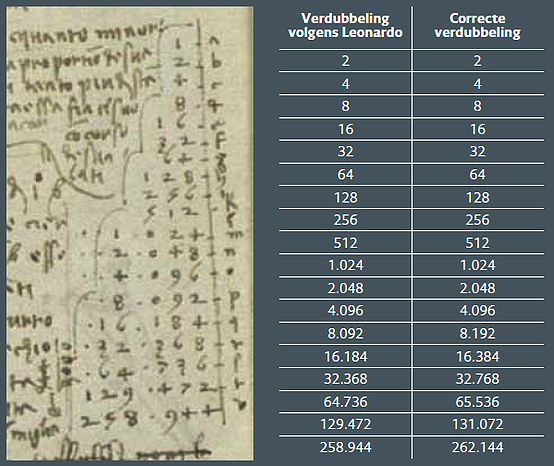
Op deze bladzijde 75R uit de Codex Madrid I nemen we de tabel aan de linkerkant van het blad onder de loep, in het midden onder de cirkel of bol. Gespiegeld zie je dat de getallen achtereenvolgende verdubbelingen van 2 betreffen. Maar Leonardo gaat in de fout bij het getal 4.096: hij verdubbelt het tot 8.092 (in plaats van 8.192). Daarna zijn de verdubbelingen opnieuw correct, als rekening wordt gehouden met de eerder gemaakte fout.
Het gaat hier om een ondubbelzinnig rekenfoutje, dat overigens niet in de schoenen van slordige kopiisten geschoven kan worden. Want het foutje staat in het (gespiegelde) handschrift van da Vinci zelf. Dat het pas nu, vijfhonderd jaar na da Vinci's dood, werd ontdekt, is wellicht een gevolg van de late ontdekking van het manuscript, nauwelijks vijftig jaar geleden.
Invoer
Een getal $n \in \mathbb{N}$.
Uitvoer
De eerste regel bevat de letter a, gevolgd door een spatie en het getal $n$. De volgende 25 regels bevatten telkens de volgende letter uit het alfabet, gevolgd door de verdubbeling van het getal op de vorige regel. In navolging van Leonardo da Vinci worden alle getallen telkens in spiegelschrift uitgeschreven, waarbij de cijfers van een getal dus in omgekeerde volgorde staan.
Voorbeeld
Invoer:
2
Uitvoer:
a 2 b 4 c 8 d 61 e 23 f 46 g 821 h 652 i 215 j 4201 k 8402 l 6904 m 2918 n 48361 o 86723 p 63556 q 270131 r 441262 s 882425 t 6758401 u 2517902 v 4034914 w 8068838 x 61277761 y 23445533 z 46880176
Bronnen
| Added by: | Peter Dawyndt |
| Date: | 2012-03-13 |
| Time limit: | 10s |
| Source limit: | 50000B |
| Memory limit: | 1536MB |
| Cluster: | Cube (Intel G860) |
| Languages: | PY_NBC |
| Resource: | None |

 RSS
RSS